SCI Publications
2011
J.C. Bennett, V. Krishnamoorthy, S. Liu, R.W. Grout, E.R. Hawkes, J.H. Chen, J. Shepherd, V. Pascucci, P.-T. Bremer.
“Feature-Based Statistical Analysis of Combustion Simulation Data,” In IEEE Transactions on Visualization and Computer Graphics, Proceedings of the 2011 IEEE Visualization Conference, Vol. 17, No. 12, pp. 1822--1831. 2011.
H. Bhatia, S. Jadhav, P.-T. Bremer, G. Chen, J.A. Levine, L.G. Nonato, V. Pascucci.
“Edge Maps: Representing Flow with Bounded Error,” In Proceedings of IEEE Pacific Visualization Symposium 2011, Hong Kong, China, Note: Won Best Paper Award!, pp. 75--82. March, 2011.
DOI: 10.1109/PACIFICVIS.2011.5742375
H. Bhatia, S. Jadhav, P.-T. Bremer, G. Chen, J.A. Levine, L.G. Nonato, V. Pascucci.
“Flow Visualization with Quantified Spatial and Temporal Errors using Edge Maps,” In IEEE Transactions on Visualization and Computer Graphics (TVCG), Vol. 18, No. 9, IEEE Society, pp. 1383--1396. 2011.
DOI: 10.1109/TVCG.2011.265
A. Cuadros-Vargas, L.G. Nonato, V. Pascucci.
“Combinatorial Laplacian Image Cloning,” In Proceedings of XXIV Sibgrapi – Conference on Graphics, Patterns and Images, pp. 236--241. 2011.
DOI: 10.1109/SIBGRAPI.2011.7
Seamless image cloning has become one of the most important editing operation for photomontage. Recent coordinate-based methods have lessened considerably the computational cost of image cloning, thus enabling interactive applications. However, those techniques still bear severe limitations as to concavities and dynamic shape deformation. In this paper we present novel methodology for image cloning that turns out to be highly efficient in terms of computational times while still being more flexible than existing techniques. Our approach builds on combinatorial Laplacian and fast Cholesky factorization to ensure interactive image manipulation, handling holes, concavities, and dynamic deformations during the cloning process. The provided experimental results show that the proposed technique outperforms existing methods in requisites such as accuracy and flexibility.
T. Etiene, L.G. Nonato, C. Scheidegger, J. Tierny, T.J. Peters, V. Pascucci, R.M. Kirby, C.T. Silva.
“Topology Verfication for Isosurface Extraction,” In IEEE Transactions on Visualization and Computer Graphics, pp. (accepted). 2011.
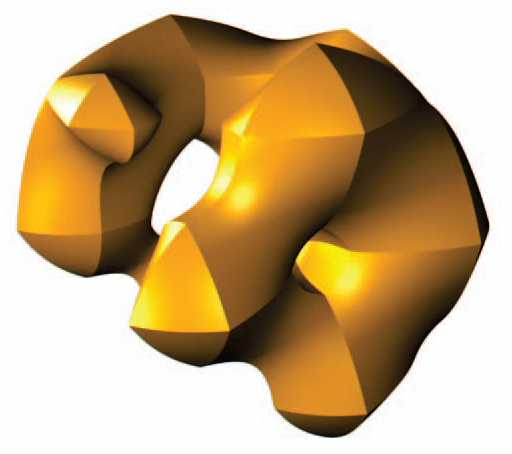
Attila Gyulassy, J.A. Levine, V. Pascucci.
“Visualization of Discrete Gradient Construction (Multimedia submission),” In Proceedings of the 27th Symposium on Computational Geometry, Paris, France, ACM, pp. 289--290. June, 2011.
DOI: 10.1145/1998196.1998241
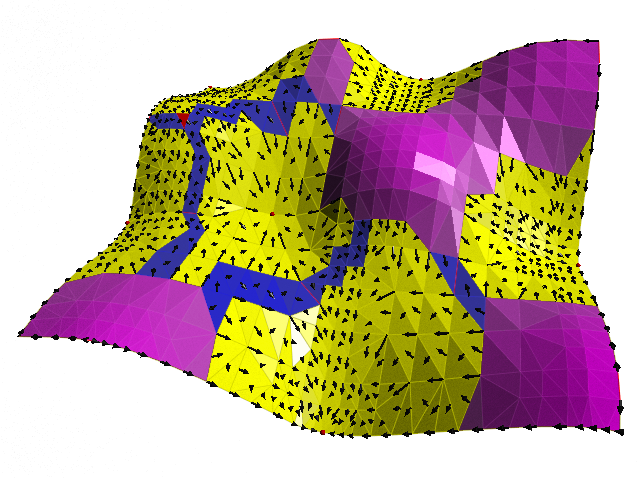
S. Jadhav, H. Bhatia, P.-T. Bremer, J.A. Levine, L.G. Nonato, V. Pascucci.
“Consistent Approximation of Local Flow Behavior for 2D Vector Fields,” In Mathematics and Visualization, Springer, pp. 141--159. Nov, 2011.
DOI: 10.1007/978-3-642-23175-9 10
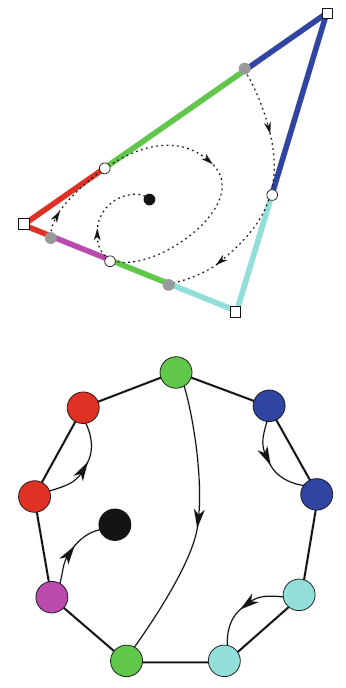
Typically, vector fields are stored as a set of sample vectors at discrete locations. Vector values at unsampled points are defined by interpolating some subset of the known sample values. In this work, we consider two-dimensional domains represented as triangular meshes with samples at all vertices, and vector values on the interior of each triangle are computed by piecewise linear interpolation.
Many of the commonly used techniques for studying properties of the vector field require integration techniques that are prone to inconsistent results. Analysis based on such inconsistent results may lead to incorrect conclusions about the data. For example, vector field visualization techniques integrate the paths of massless particles (streamlines) in the flow or advect a texture using line integral convolution (LIC). Techniques like computation of the topological skeleton of a vector field, require integrating separatrices, which are streamlines that asymptotically bound regions where the flow behaves differently. Since these integrations may lead to compound numerical errors, the computed streamlines may intersect, violating some of their fundamental properties such as being pairwise disjoint. Detecting these computational artifacts to allow further analysis to proceed normally remains a significant challenge.
S. Kumar, V. Vishwanath, P. Carns, B. Summa, G. Scorzelli, V. Pascucci, R. Ross, J. Chen, H. Kolla, R. Grout.
“PIDX: Efficient Parallel I/O for Multi-resolution Multi-dimensional Scientific Datasets,” In Proceedings of The IEEE International Conference on Cluster Computing, pp. 103--111. September, 2011.
T. Peterka, R. Ross, A. Gyulassy, V. Pascucci, W. Kendall, H.-W. Shen, T.-Y. Lee, A. Chaudhuri.
“Scalable Parallel Building Blocks for Custom Data Analysis,” In Proceedings of the 2011 IEEE Symposium on Large-Scale Data Analysis and Visualization (LDAV), pp. 105--112. October, 2011.
DOI: 10.1109/LDAV.2011.6092324
We present a set of building blocks that provide scalable data movement capability to computational scientists and visualization researchers for writing their own parallel analysis. The set includes scalable tools for domain decomposition, process assignment, parallel I/O, global reduction, and local neighborhood communicationtasks that are common across many analysis applications. The global reduction is performed with a new algorithm, described in this paper, that efficiently merges blocks of analysis results into a smaller number of larger blocks. The merging is configurable in the number of blocks that are reduced in each round, the number of rounds, and the total number of resulting blocks. We highlight the use of our library in two analysis applications: parallel streamline generation and parallel Morse-Smale topological analysis. The first case uses an existing local neighborhood communication algorithm, whereas the latter uses the new merge algorithm.
S. Philip, B. Summa, P.-T. Bremer, and V. Pascucci.
“Parallel Gradient Domain Processing of Massive Images,” In Proceedings of the 2011 Eurographics Symposium on Parallel Graphics and Visualization, pp. 11--19. 2011.

S. Philip, B. Summa, P-T Bremer, V. Pascucci.
“Hybrid CPU-GPU Solver for Gradient Domain Processing of Massive Images,” In Proceedings of 2011 International Conference on Parallel and Distributed Systems (ICPADS), pp. 244--251. 2011.

M. Schulz, J.A. Levine, P.-T. Bremer, T. Gamblin, V. Pascucci.
“Interpreting Performance Data Across Intuitive Domains,” In International Conference on Parallel Processing, Taipei, Taiwan, IEEE, pp. 206--215. 2011.
DOI: 10.1109/ICPP.2011.60
B. Summa, G. Scorzelli, M. Jiang, P.-T. Bremer, V. Pascucci.
“Interactive Editing of Massive Imagery Made Simple: Turning Atlanta into Atlantis,” In ACM Transactions on Graphics, Vol. 30, No. 2, pp. 7:1--7:13. April, 2011.
DOI: 10.1145/1944846.1944847
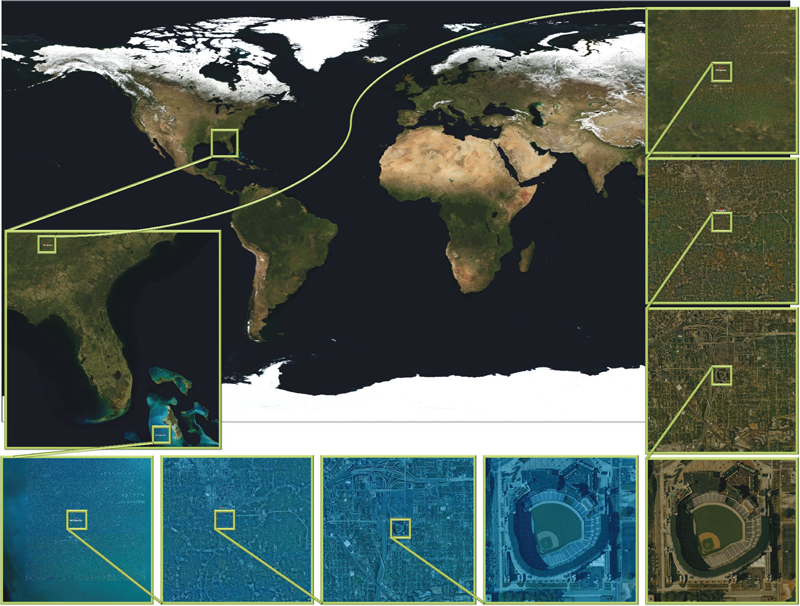
This article presents a simple framework for progressive processing of high-resolution images with minimal resources. We demonstrate this framework's effectiveness by implementing an adaptive, multi-resolution solver for gradient-based image processing that, for the first time, is capable of handling gigapixel imagery in real time. With our system, artists can use commodity hardware to interactively edit massive imagery and apply complex operators, such as seamless cloning, panorama stitching, and tone mapping.
We introduce a progressive Poisson solver that processes images in a purely coarse-to-fine manner, providing near instantaneous global approximations for interactive display (see Figure 1). We also allow for data-driven adaptive refinements to locally emulate the effects of a global solution. These techniques, combined with a fast, cache-friendly data access mechanism, allow the user to interactively explore and edit massive imagery, with the illusion of having a full solution at hand. In particular, we demonstrate the interactive modification of gigapixel panoramas that previously required extensive offline processing. Even with massive satellite images surpassing a hundred gigapixels in size, we enable repeated interactive editing in a dynamically changing environment. Images at these scales are significantly beyond the purview of previous methods yet are processed interactively using our techniques. Finally our system provides a robust and scalable out-of-core solver that consistently offers high-quality solutions while maintaining strict control over system resources.
D. Thompson, J.A. Levine, J.C. Bennett, P.-T. Bremer, A. Gyulassy, V. Pascucci, P.P. Pebay.
“Analysis of Large-Scale Scalar Data Using Hixels,” In Proceedings of the 2011 IEEE Symposium on Large-Scale Data Analysis and Visualization (LDAV), Providence, RI, pp. 23--30. 2011.
DOI: 10.1109/LDAV.2011.6092313
H.T. Vo, J. Bronson, B. Summa, J.L.D. Comba, J. Freire, B. Howe, V. Pascucci, C.T. Silva.
“Parallel Visualization on Large Clusters using MapReduce,” SCI Technical Report, No. UUSCI-2011-002, SCI Institute, University of Utah, 2011.
H.T. Vo, J. Bronson, B. Summa, J.L.D. Comba, J. Freire, B. Howe, V. Pascucci, C.T. Silva.
“Parallel Visualization on Large Clusters using MapReduce,” In Proceedings of the 2011 IEEE Symposium on Large-Scale Data Analysis and Visualization (LDAV), pp. 81--88. 2011.
Keywords: MapReduce, Hadoop, cloud computing, large meshes, volume rendering, gigapixels
H.T. Vo, C.T. Silva, L.F. Scheidegger, V. Pascucci.
“Simple and Efficient Mesh Layout with Space-Filling Curves,” In Journal of Graphics, GPU, and Game Tools, pp. 25--39. 2011.
ISSN: 2151-237X
Bei Wang, B. Summa, V. Pascucci, M. Vejdemo-Johansson.
“Branching and Circular Features in High Dimensional Data,” SCI Technical Report, No. UUSCI-2011-005, SCI Institute, University of Utah, 2011.
Bei Wang, B. Summa, V. Pascucci, M. Vejdemo-Johansson.
“Branching and Circular Features in High Dimensional Data,” In IEEE Transactions of Visualization and Computer Graphics (TVCG), Vol. 17, No. 12, pp. 1902--1911. 2011.
DOI: 10.1109/TVCG.2011.177
PubMed ID: 22034307
Keywords: Dimensionality reduction, circular coordinates, visualization, topological analysis
S. Williams, M. Petersen, P.-T. Bremer, M. Hecht, V. Pascucci, J. Ahrens, M. Hlawitschka, B. Hamann.
“Adaptive Extraction and Quantification of Geophysical Vortices,” In IEEE Transactions on Visualization and Computer Graphics, Proceedings of the 2011 IEEE Visualization Conference, Vol. 17, No. 12, pp. 2088--2095. 2011.
Page 7 of 13
